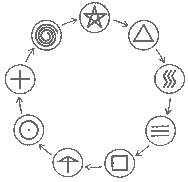
Jot down your telephone number. Scramble the digits in any way you please to form a new number. Take the smaller number away from the larger one. Add all the digits in your answer. Now place you finger on the star at the top of the magic circle below and count clockwise (Star -1, triangle -2 etc.) until you reach the total you calculated when you added the digits together.

The count will end on the spiral. Why?
This is an easy one, once you know the answer. It is best to try it out with a bit of string.
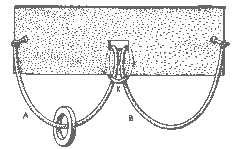
Simply, how do you get the ring from side A to side B if it wont fit through the hole in the wood and you cannot undo any knots?
Five men and a monkey were shipwrecked on a desert island so they spent the first day gathering coconuts for food. They put their coconuts in a large pile.
That night, while they were asleep, one man woke up and thought that there might be an arguement about dividing the coconuts in the morning, so he decided to take his share now. He divided the coconuts fairly into five piles. There was one coconut left over, so he gave it to the monkey. He hid his share and put the rest back together in a single pile.
By and by the second man woke up and did the same thing. He also had one left over and gave it to the monkey. All five men did the same thing, one after the other. Each took a fifth share of the pile when he woke, had one left over which he gave to the monkey and returned to sleep.
In the morning they divided up the pile into five equal shares. (As everyone felt guilty, no one complained that the pile had diminished in the night!).
How many coconuts did they collect in the first place?
This is my favorite one.
A man is shipwrecked in a storm near an island on which are known to live two tribes. The blue eyed tribe always speak the truth and the brown eyed tribe always lie.
As he is washed towards the shore clutching a piece of wood the man is worried that there may be rocks which will dash him to pieces. He can just make out three figures on the beach and decides to ask them if ithe beach is safe but first he must know whether they tell truth or lies.
He calls out through the storm, "Do you have blue eyes or brown eyes?" The first figure shoutsc back but his reply is lost in the wind. The second figure says, "He says that his eyes are blue and so are mine". The third figure says, "No, they lie, Their eyes are brown and mine are blue".
So who is telling the truth?
A King collects a bag of silver coins from each of his twelve of his Lords. But his spies tell him that one of the Lords has cheated him by supplying silver coins that are exactly 1 gram underweight. Unfortunately, the spy could not say which Lord was cheating the King.
As is the custom in this kind of problem the King a pair of magic scales and although they were very accurate they could only ever be used to make ONE measurement in grams.
How could the King be sure to find out which Lord was guilty if he only has one chance to weigh the coins?
A fisherman rows out to the centre of a pond in a small rowing boat. As he launches the boat it will of course displace its own weight (plus his weight) in water and consequently the level of the water in the pond will rise very slightly.
When he reaches the centre, he takes his anchor and throws it into the water. As it settles on the pond's bottom, what difference does this make to the level of the water in the pond, if any?